For many readers, the phrase "utility maximization" may conjure up undesirable flashbacks to their principles of economics course in college. Any efforts to reconnect with those ideas would also be stymied by online searches that mostly turn up equation-heavy treatises with very little intuition. But when it comes to retirement planning, this concept is much more important and less boring than it sounds.
This is illustrated in the new research article, "Determining Optimal Withdrawal Rates: An Economic Approach," by Duncan Williams and Michael Finke of Texas Tech University. The article appears in the Fall 2011 issue of the Retirement Management Journal, and it won the journal's Academic Thought Leadership Award. Williams and Finke find that an aggressive retiree with a $1 million nest egg and a guaranteed Social Security income base of $20,000, could actually maximize their utility with a 7 percent withdrawal rate. So much for the 4 percent rule!
I am not usually one to advocate high withdrawal rates in retirement. In fact, I have an article in that same journal issue which summarizes my concerns about the sustainability of 4 percent withdrawals for retirees in recent years. Incorporating some of my concerns, such as whether we should be assuming that future market returns will be as high as witnessed in the past, might reduce the utility maximizing withdrawal rates a bit. However, it would not detract from the overall point of their article, which is to show in a systematic manner when retirees might be willing to accept a rather high portfolio failure rate. Perhaps there has been too much emphasis in retirement withdrawal rate research on keeping failure rates low.
To understand the context behind their utility maximization approach, let us first briefly review how financial planners have examined the issue of retirement withdrawal rates. The common approach looks at shortfall risk, or the probability of running out of wealth within a specified time period for a particular inflation-adjusted withdrawal rate and asset allocation strategy. William Bengen's seminal 1994 article started things off by demonstrating that from overlapping 30-year periods of the historical data since 1926, a 4 percent withdrawal rate never failed in providing the necessary inflation-adjusted withdrawal amounts. Monte Carlo simulations, which provide more potential scenarios than our relatively short historical period, generally confirm that the failure rate for the 4 percent rule is under 10 percent for this range of stock allocations. Using Ibbotson Associates' data on total returns for large-capitalization stocks and long-term government bonds since 1926, the following figure shows the relationship between asset allocation, withdrawal rates, and failure rates for the most commonly used metric of a 30-year retirement horizon.
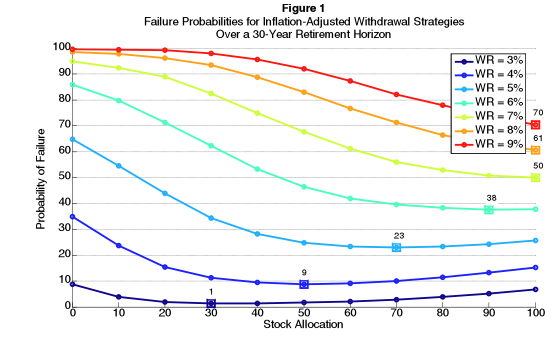
But this, actually, is a rather clumsy way to consider a retiree's attitudes toward risk. First of all, what is an acceptable failure rate for retirees? Rory Terry argued in the May 2003 Journal of Financial Planning that due to the high costs of failure, even a 1 percent failure rate sounds exceedingly high. On the other hand, in the April 2011 Journal of Financial Planning, Philip Cooley, Carl Hubbard and Daniel Walz suggest that retirees might accept a 25 percent failure rate with the understanding that they may need to make mid-course adjustments to reduce their spending. Retirees are left to choose their withdrawal rate and asset allocation based on what they deem to be an acceptable failure rate.
About this whole matter of failure rates, though, Williams and Finke cleverly point out that it is not really the failure rate that matters for retirees so much as the percentage of time at the end of retirement that retirees should expect to endure not having any remaining wealth. In that regard, we cannot really decide on an appropriate failure rate without knowing whether retirees are setting themselves up for one or 10 years of life without any wealth, and also without considering how much retirees can rely on other sources of guaranteed income, such as Social Security and employer defined-benefit pensions, in the event that their other financial assets are gone. More guaranteed income and a shorter period of failure at the end of life both suggest higher acceptable failure rates due to the lessened damage from wealth depletion.
Utility is important because it provides a systematic way to evaluate how retirees can make decisions about the appropriate withdrawal rate and asset allocation strategy for their personal retirement situation. It helps prospective retirees to find the appropriate balance among a lot of complicated tradeoffs. On the one hand, if retirees increase their withdrawal rate they can enjoy heightened spending early in retirement, but this also increases the probability of running out of wealth later in retirement. Spend too little, though, and retirees risk missing opportunities to spend from their hard-earned savings. Increasing the stock allocation will generally reduce the failure rates when the withdrawal rate is large enough, but at the cost of increasing the magnitude of those failures as retirees spend more years without wealth in the cases when failures happen.
Utility also incorporates the idea of diminishing marginal returns, which is that increasing income levels do not increase happiness at a constant rate. An extra $5,000 of income will be more valuable to someone living on $10,000 than to someone living on $100,000. Some people, though, are more aggressive than others in terms of their willingness to accept larger losses for the prospects of potentially enjoying larger gains. Utility accounts for this, as it allows risk averse individuals to place additional emphasis on avoiding bad outcomes even though it means spending less during the rest of retirement.
So what do Williams and Finke find when applying utility analysis to the issue of retirement planning? I have replicated their methodology to illustrate several of the key points from their new research. The following figures show the "certainty equivalence" values, which are the lowest fixed-income levels a retiree would be willing to accept to avoid the uncertainty associated with spending more while they still have remaining wealth and spending less when their wealth is gone. Williams and Finke use mortality rates to calculate the percentage of one's life expected to be spent without any remaining wealth. Consider someone with a guaranteed income of $20,000. Using a 5 percent withdrawal rate with a 50 percent stock allocation, Williams and Finke might find, for instance, that such a strategy will provide wealth, on average, for 98 percent of one's remaining life (with a $1 million nest egg this would mean a total real spending amount of $70,000 in these years) and wealth will be depleted, on average, for 2 percent of one's life (allowing for real spending of $20,000 in those years). The "certainty equivalence" is the constant real spending amount with 100 percent certainty that the retiree would accept to avoid the volatility of spending amounts just described, and a more risk averse retiree would accept a lower spending amount to avoid this uncertainty. These values are calculated using a rather complex-looking formula that includes the spending amounts when wealth does and does not remain, the probabilities for these two outcomes, and a measure of the retiree's risk aversion. Whichever strategy provides the largest certainty equivalence is the one that maximizes the retiree's utility.

Figure 2 shows the case for a risk-tolerant male retiring at age 65, who has guaranteed inflation-adjusted income sources of $20,000 (Social Security, for instance), and a $1 million nest egg. Far from the 4 percent rule, the figure shows how this retiree can maximize his utility using a 7 percent withdrawal rate with a 70 percent stock allocation. Rather shockingly, based on the traditional shortfall risk approach, this strategy actually would lead to a 57 percent chance of running out of wealth within 30 years. This, indeed, is the most surprising and thought-provoking insight coming from Williams and Finke's research. Acceptable failure rates might be much higher than we ever imagined. Calls for low failure rates may not have properly accounted for the risk aversion or the other sources of income available for retirees who may be willing to risk higher failure for the opportunity to spend more earlier on in their retirements.
This new research does not always call for such high withdrawal rates. The scenario shown in Figure 3 is the same as in Figure 2, except that now we are investigating the case for a more conservative and risk-averse 65-year old male. For this retiree, a 4 percent withdrawal rate with a 40 percent stock allocation is utility maximizing. This particular result is close to what is generally recommended in traditional shortfall risk studies.
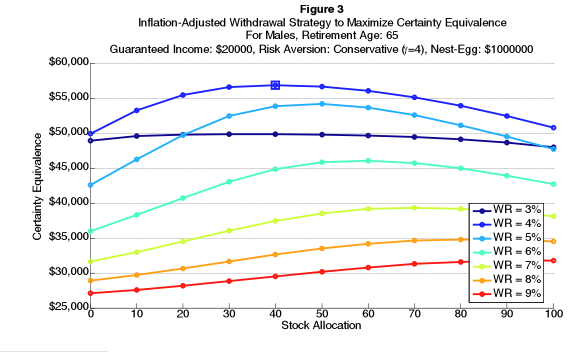
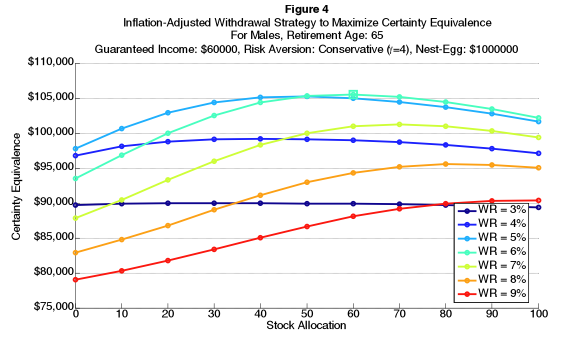
But still, that is not the whole story. Figure 4 considers the same risk averse retiree as Figure 3, but now he has access to guaranteed income of $60,000 (perhaps an employer defined-benefit pension as well) instead of $20,000. The extra guaranteed income reduces the impact of running out of wealth, and now even this conservative retiree finds that a 6 percent withdrawal rate with a 60 percent stock allocation is what will maximize his utility. Looking back to Figure 1 again, the implication is that even this conservative retiree is willing to accept a 42 percent failure rate because of the extra guaranteed income he has at his disposal.
While the new research provides an initial exploration for the case of constant inflation-adjusted withdrawal amounts, I look forward to seeing further research with this methodology to consider many other questions. These tools are useful for guiding decisions about annuitizing part of a retiree's assets. They are also useful for comparing the many competing variable withdrawal rate strategies, such as constant percentage of portfolio withdrawals, William Bengen's ceiling-and-floor approach, and Jonathan Guyton's decision rules. As well, utility maximization should be applied to the entire lifetime, since using lower withdrawal rates to reduce the chances for failure also implies a need to save more and consume less during one's working years. It is time to dust off those old textbooks and see what sorts of insights utility maximization may provide to strengthen the retirement planning process.
Wade D. Pfau, Ph.D., an associate professor at the National Graduate Institute for Policy Studies in Tokyo, Japan, holds a doctorate in economics from Princeton University. You can read more about his retirement planning research in an article by advisor Dan Moisand by clicking here. http://www.fa-mag.com/online-extras/7006-a-safe-retirement-savings-rate.html








