Why are some financial advisory firms more successful than others? Why do some become better over time while others stagnate, eventually fading into oblivion? Is there a single secret of success for these businesses?
The answers to these questions are usually complex, feature lists and go something like this: Successful advisory companies possess, employ and benefit from a favorable mix of vision, strategy, execution, skill, expertise, market conditions, leadership and management, capital, innovation, external relationships, timing and luck.
While answers like this can be helpful, at least as a starting point, they typically omit the most important of all prerequisites for success—a key commonality that links and empowers all successful advisory organizations: They are full of people who have placed their trust in one another and therefore can cooperate with maximum efficacy.
Sound a bit New Age to you? Like something you might hear at a wellness seminar over a quinoa and kale salad (with the dressing on the side)? Well, it’s actually an inarguable fact based on math and logic. Certainly, we financial advisors driving for success can respect math and logic, can’t we?
To grasp this concept, let’s travel back in time and visit 18th century philosopher Jean-Jacques Rousseau. Rousseau imagined a stag hunt. A group of hunters have tracked and cornered a large stag and, if we assume they are patient and cooperate, should be successful in their hunt, providing an ample food source for themselves and their families for several days. If, however, they do not cooperate or are noticed by the deer, they will lose it and go hungry.
They lie in wait for many hours when, suddenly, some hares wander right in front of them. If one of the hunters goes after a hare—an easier target that will feed that particular hunter and their family for a day—the stag will surely notice and flee, causing the hunters who did not go for a hare to go hungry.
Consider this dilemma. Each hunter must individually consider the risk that any one of their companions might selfishly go for a hare, as any defectors know that at least they will eat even if the other stag hunters go hungry. What would you do if you were one of these hunters?
Enter John Forbes Nash Jr., the 20th century mathematician whose story is told in the book and movie A Beautiful Mind. Nash, who substantially advanced the field of game theory—the mathematical study of strategic interactions among rational decision-makers—discovered the concept of “Nash equilibria.” These occur when “players” (decision-makers) each make their best decision taking into account the decisions of the other players, which remain unchanged.
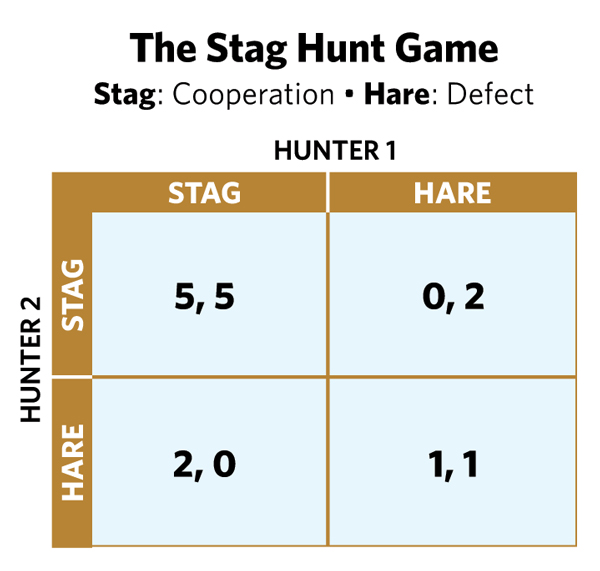
In this table, assuming there are only two hunters, both will have five days of meat if they cooperate in hunting the stag. If they both go for the hares, they will each have one day of meat. If, however, one goes for the stag while the other goes for the hares, then the latter will have two days of meat, and the former will have none. The 5,5 and 1,1 results are both Nash equilibria, though the 5,5 result is obviously better for both hunters.








